Integral calculus is a branch of calculus that revolves around the study of integrals and their applications. Finding integral is the inverse operation of differentiation. Integrals and differentials are closely related to each other by the fundamental theorem of calculus. They jointly serve as the building blocks of mathematical analysis.
Integral is a powerful mathematical tool for calculating the area under a curve and the volume of a solid. This article will explore the definition of integral with its types. We will learn different methods to calculate integrals with the help of examples.
What is an Integral?
An integral is a mathematical concept that is used to determine the net effect of a continuously changing quantity. Its primary purpose is to find the area under a curve in a two-dimensional plane and the volume of a three-dimensional object. Integrals can be thought of as the reverse process of differentiation.
An integral is like adding up tiny slices of a function to find the total value. It has many practical applications in engineering and economics.
Types of Integral
There are two main types of integrals in calculus:
- Definite Integral
- Indefinite Integral
These integrals serve different purposes and have distinct notations.
Definite Integral:
This type of integral is used to find the exact numerical value of the accumulated quantity between two specific points on a curve or within a specific interval. Real numbers are the result of definite integrals. The definite integral may be represented as:
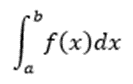
Where a is lower and b is the upper limit of integration (a < b).
Indefinite Integral (antiderivative):
This type of integral is used to find a function whose derivative is the original function we are integrating. It includes a constant of integration (often denoted as +C) because there can be multiple functions with the same derivative. It is denoted by
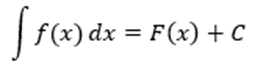
Where,
- ∫ represents the process of integration.
- f(x) is the function that we want to integrate.
- dx denotes the variable with respect to which we are integrating.
- F(x) is the anti-derivative of f(x)
- C shows the constant of integration.
The Fundamental Theorems of Calculus
It establishes a link between integration and differentiation in calculus. Let’s explore two parts of this theorem.
First Fundamental Theorem of Calculus (FTC 1):
If a continuous function f(x) is defined on a closed interval [a, b] and we define a new function F(x) as follows:
F(x) = ∫a b f(t) dt
Then the derivative of F(x) with respect to x is equal to the original function f(x):
F’(x) = f(x)
Second Fundamental Theorem of Calculus (FTC 2):
The Second Fundamental Theorem of Calculus provides a shortcut for calculating definite integrals by finding the difference between the antiderivative evaluated at the upper and lower bounds of the interval.
∫a b f(x) dx = F (b) – F (a)
Integral formulas
Here are some commonly used integral formulas in calculus:
- Power Rule
∫ xn dx = ( xn+1 / (n + 1)) + C (where ‘n’ is any real number except -1)
- Integral for Constant
∫ a dx = ax + C (where ‘a’ is any constant)
- Integral formula of Exponential function
∫ ex dx = ex + C
- Integral formula for Natural Logarithm
∫ (1/x) dx = ln|x| + C
∫ ax dx = ax/ln(a) + C
- Integral formulas for Trigonometric function
∫ Sin(x) dx = – cos(x) + C
∫ Cos(x) dx = Sin (x) + C
∫ Tan(x) dx = ln|sec (x)| + C = – ln|cos(x)| + C
∫ Sec(x) dx = ln|tan(x) + sec (x)| + C
∫ Csc(x) dx = ln|csc(x) – cot (x)| + C
∫ Cot(x) dx = ln|sin(x)| + C
∫ Sec2(x) dx = tan (x) + C
∫ Csc2(x) dx = – cot (x) + C
∫ Sec(x) tan(x) dx = sec (x) + C
∫ Csc(x) cot(x) dx = – csc (x) + C
Techniques of Integration
There are several ways used to find the integration of function in calculus. Some commonly used methods are here:
Solve Integral by Substitution Method
Substitution involves replacing a complicated expression with a simpler one to facilitate integration. This technique is especially useful for trigonometric and exponential functions.
If t is a function of u then
t’ = dt/du
∫ f(t)t’ du = ∫ f(t) dt, where t = g(u).
Integration by Parts
Integration by parts is a method to integrate the product of two functions by applying the formula:
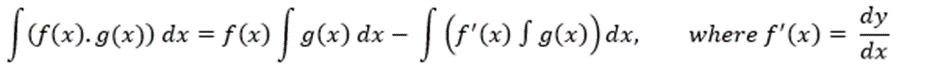
Partial Fractions
Partial fractions are used to simplify complex rational functions by breaking them down into simpler fractions.
Online Integral calculator
Using an integral calculator can save time and help avoid calculation errors, making it a valuable tool when working with complex integrals in calculus. However, it’s essential to understand the underlying calculus concepts to effectively use these calculators and interpret the results.
Examples of Integral with Solution
Here are some examples to learn how to solve integral problems.
Example 1:
Compute ∫ (5x4 + x3 + 4x +1) dx
Solution
∫ (5x4 + x3 + 4x + 1) dx
= 5 ∫ x4 dx + ∫ x3 dx + 4∫ x dx + ∫1dx
Apply the Power rule
= 5(x4 +1 / 4 + 1) + (x3+1 / 3 + 1) + 4(x1+1 / 1 + 1) + x + C
= 5(x5/5) + (x4/4) + 4(x2 /2) + x + C
= (x5) + (x4/4) + 2(x2) + x + C
Thus, the integration of the given function is (x5) + (x4/4) + 2(x2) + x + C
An integral calculator by MeraCalculator is an alternate way for solving integral problems to ease up calculations.
Example 2:
Evaluate ∫0𝜋 tan2(x) dx
Solution
∫0𝜋 tan2(x) dx
Use a trigonometric identity to simplify the integrand
∴ tan2(x) = sec2(x) – 1
∫0𝜋 tan2(x) dx = ∫0𝜋 (sec2(x) – 1) dx
= ∫0𝜋 sec2(x) dx – ∫0𝜋 1 dx
= [tan (x)] 0𝜋 – [x] 0𝜋
= [tan (𝜋) – tan (0)]– [𝜋 – 0]
= 0 – 𝜋
= – 𝜋
Thus, ∫0𝜋 tan2 (x) dx = – 𝜋
Conclusion
In this article, we have covered the concept of integral calculus, including its definition and types. We have discussed fundamental theorems and methods for complex integrals. Examples have included both definite and indefinite integrals. After reading, you will have the skills to solve any integration problem.
